Zur Beschreibungsseite auf Commons

Datei:Topological space examples.svg

aus Wikipedia, der freien Enzyklopädie

Originaldatei ‎(SVG-Datei, Basisgröße: 360 × 319 Pixel, Dateigröße: 12 KB)

Commons-logo.svg

Diese Datei und die Informationen unter dem roten Trennstrich werden aus dem zentralen Medienarchiv Wikimedia Commons eingebunden.

Zur Beschreibungsseite auf Commons

Beschreibung

Beschreibung
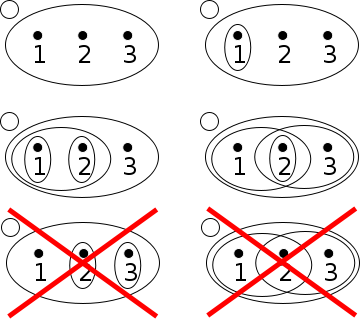
English: Examples and non-examples of topological spaces, based roughly on Figures 12.1 and 12.2 from Munkres' Introduction to Topology. The 6 examples are subsets of the power set of {1,2,3}, with the small circle in the upper left of each denoting the empty set, and in reading order they are:
  • {{}, {1,2,3}} (the trivial topology)
  • {{}, {1}, {1,2,3}}
  • {{}, {1}, {2}, {1,2}, {1,2,3}}
  • {{}, {2}, {1,2}, {2,3}, {1,2,3}}
  • {{}, {2}, {3}, {1,2,3}} (not a topology, because {2} and {3} are in the topology but their union {2,3} is not)
  • {{}, {1,2}, {2,3}, {1,2,3}} (not a topology, because {1,2} and {2,3} are in the topology but their intersection {2} is not)
Deutsch: Beispiele und Gegenspiele zu topologischen Räumen, die grob den Abbildungen 12.1 und 12.2 aus dem Buch Introduction to Topology von Munkres entsprechen. Die sech Beispiele sind Teilmenge der Potenzmenge von {1,2,3}. Der kleine Kreis in den Beispielen oben links bezeichnet die leere Menge. Im Detail lauten die Beispiele dann:
  • {{}, {1,2,3}} (triviale Topologie)
  • {{}, {1}, {1,2,3}}
  • {{}, {1}, {2}, {1,2}, {1,2,3}}
  • {{}, {2}, {1,2}, {2,3}, {1,2,3}}
  • {{}, {2}, {3}, {1,2,3}} (kein topologischer Raum, da die Mengen {2} und {3} zur Topologie gehören, aber ihre Vereinigung {2,3} nicht)
  • {{}, {1,2}, {2,3}, {1,2,3}} (kein topologischer Raum, da die Mengen {1,2} und {2,3} zur Topologie gehören, aber der Schnitt {2} der beiden nicht)
Español: Ejemplos y contraejemplos de espacios topológicos basados, fundamentalmente, en las Figuras 12.1 y 12.2 del libro "Introduction to Topology" de Munkres. Los seis ejemplos son subconjuntos del conjunto potencia {1,2,3}, donde el círculo pequeño en la esquina superior izquierda de cada figura representa el conjunto vacío, y que en orden de lectura son
  • {{}, {1,2,3}} (topología trivial)
  • {{}, {1}, {1,2,3}}
  • {{}, {1}, {2}, {1,2}, {1,2,3}}
  • {{}, {2}, {1,2}, {2,3}, {1,2,3}}
  • {{}, {2}, {3}, {1,2,3}} (no es topológico porque {2} y {3} se encuentran en la topología, pero su unión {2,3} no)
  • {{}, {1,2}, {2,3}, {1,2,3}} (no es topológico porque {1,2} y {2,3} se encuentran en la topología, pero su intersección {2} no)
Datum
Quelle Eigenes Werk
Urheber Dcoetzee

Lizenz

Public domain Ich, der Urheberrechtsinhaber dieses Werkes, veröffentliche es als gemeinfrei. Dies gilt weltweit.
In manchen Staaten könnte dies rechtlich nicht möglich sein. Sofern dies der Fall ist:
Ich gewähre jedem das bedingungslose Recht, dieses Werk für jedweden Zweck zu nutzen, es sei denn, Bedingungen sind gesetzlich erforderlich.

Kurzbeschreibungen

Ergänze eine einzeilige Erklärung, was diese Datei darstellt.

In dieser Datei abgebildete Objekte

Motiv

Dateigröße

12.644 Byte

Höhe

319 Pixel

Breite

360 Pixel

MIME-Typ

image/svg+xml

Prüfsumme

5095c19ae50d1c3f6b179a7253ed695e87c4b36a

Bestimmungsmethode: SHA-1

Dateiversionen

Klicke auf einen Zeitpunkt, um diese Version zu laden.

Version vomVorschaubildMaßeBenutzerKommentar
aktuell06:54, 26. Mai 2008Vorschaubild der Version vom 06:54, 26. Mai 2008360 × 319 (12 KB)wikimediacommons>Dcoetzee{{Information |Description=Examples and non-examples of topological spaces, based roughly on Figures 12.1 and 12.2 from Munkres' ''Introduction to Topology''. The 6 examples are subsets of the power set of {1,2,3}, with the small circle in the upper left

Die folgende Seite verwendet diese Datei:

Abgerufen von „https://de.wikiup.org/wiki/Datei:Topological_space_examples.svg“